- PIs: Calabrese, Delfino, Gambassi, Mussardo
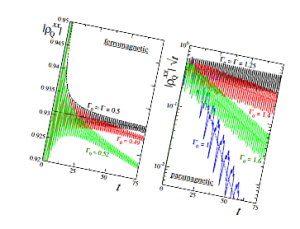
Suppose that an extended quantum system, e.g. one made of optically trapped cold atoms, is subjected to an abrupt change of the parameters of its Hamiltonian and, after that, evolves according to the unitary dynamics of the new Hamiltonian: does it thermalize or not? Will it have memory of its initial state? How does this thermalization occur? Is there a difference between integrable and non-integrable dynamics, as recent experiments seem to suggest? For integrable systems, what is the correct statistical ensemble that describes the infinite-time equilibrium situation? Is there any emergent universality? A whole lot of physics, as it emerges from the alluring fast developing field of quantum systems out of equilibrium, is calling for a proper analysis.
Developing a profound understanding of the connection between ergodicity, integrability and thermalization in quantum systems, and exploring the great interdisciplinary potentialities of this research area, is an important target of our research that deals with exactly solved lattice models, quantum spin chains, cold atom systems and quantum field theory. Particular emphasis is given to quantum integrable dynamics of such systems and its breaking. Advanced methods of analysis include Conformal Field Theory, Form Factors, Bethe Ansatz, Boundary Field Theory, Keldysh formalism, Random Matrices, Semi-classical Field Theories and Truncated Conformal Space Approach.
At the same time, attempts to understand interacting disordered quantum systems in terms of their excitations, energy and particle transport, relaxation dynamics and thermalization have been the focus of much attention recently. In quantum systems, strong disorder may lead to non-ergodicity and entail permanent out-of-equilibrium and glassy behavior. A particularly interesting question concerns the problem of understanding how such non-ergodicity arises close to disorder-tuned quantum phase transitions between conducting and insulating phases. Recent results suggest a rich phenomenology of spatial and spectral properties of the relevant excitations and ground states.
We address the interplay of interactions, glassiness, localization, and superfluidity from many different angles, trying to understand the transition from non-ergodic to ergodic quantum dynamics in strongly disordered many body systems.
Collaborators: Scardicchio

